What is an Artefact Design?

Pawel Garbacz
Faculty of Philosophy
The John Paul II Catholic University of Lublin

Abstract

The paper contains a first order formal theory pertaining to artefact designs, designs which are construed as the results of designing activities. The theory is based on a minimal ontology of states of affairs and it is inspired by the ideas of the Polish philosopher Roman Ingarden. After differentiating the philosophical notion of design from the engineering notion of design specifications, I then go on to argue that the philosophical category of artefact designs may be compared with Ingarden’s category of intentional states of affairs. At least some artefacts are found to be determined by more than one design. I also show how this ontological framework allows for the distinction between artefact tokens and artefact types. That leads to a proposal on how to define a criterion of identity for artefact types. The proposed theory serves as a basis both for a better understanding of what artefacts are and for the construction of computer-readable models of design specifications.

Keywords: artefact, design, ontology, representation

The category known as design is a crucial notion in engineering science. In engineering and in analyses of this notion the focus tends to lie predominantly on the process of designing, rather than on the results or products of design. Engineering theories of design inform us about the stages and principles of design, but they are not so explicit when it comes to the nature of the design specification itself. ¹ Needless to say, philosophical reflections on the ontic structure of designing and its products are even scarcer. It might be argued that the lack of analyses of design products hinders ontological investigation into the nature of artefacts both in engineering and philosophy since it is the design specifications that determines, or at least co-determines, the intentional aspects thereof. Given the suitable level of granularity of our descriptions of artefacts it may be asserted that they are what they are due to their design specifications. In this paper I will analyse the products of designing in order to contribute to the resolving of philosophical puzzles about the identity of artefacts and to aid the engineering search for adequate representations of design data. What I have in mind is the approaches in engineering design that resort to the idea of engineering ontologies that can provide the foundations for computer-readable representations of design specifications (e.g. Alberts (1994), Gruber and Olsen (1994), Chandrasekaran et al. (1999), Kitamura et al. (2002), to name but a few).

The principal aim of this paper is to give an ontological account of designs that are construed as products of design activity. It is an essay on slightly revisionary metaphysics. In other words, instead of attempting to define the standard meanings of the defined notions, I will suggest a new framework within which artefacts and their designs can be considered. By approximating some fundamental intuitions on the production process, the framework will to be evaluated in the light of its consistency and explanatory power. The resulting theory will be rendered in first-order predicate language. The ontological foundation for this enterprise remains the philosophy of Roman Ingarden. Provided that this account is adequate, it will provide the grounds both for a better understanding of what artefacts are and for the construction of computer-readable models of design specifications. The main results comprise:

• separation of different modes of artefact representations,
• distinction between the engineering and philosophical notion of design,
• conceptual framework to explicate the variable granularity of designs,
• distinction between artefact tokens and the artefact types the account suggests against a criterion of identity for artefact types.

For the sake of simplicity, the temporal aspects of artefact designs will be ignored. This constraint will force me to focus not so much on the process of designing as on the product of designing. All temporal indices in the respective definitions and theorems will therefore also be omitted. The constraint does not mean, however, that artefact designs will be construed in such a way as to exclude the behavioural or functional descriptions of artefacts.

The theory I expose here is a rightful part of a more comprehensive approach known as the four-dimensional ontology of artefacts. In this ontology, artefacts are characterised according to their purposes, designs, the background knowledge in which the designs are embedded and the instructions of use. The details of this approach may be found in ( Garbacz 2004 ). Here I will only briefly note that my view of artefacts is convergent with the Constitution View, expressed in this issue by Lynne Baker, which states that artefacts are intention-dependent objects that are constituted by physical entities.

1. Artefacts and designs

When an engineer speaks about design, he or she usually has in mind some kind of designing activity. The standard view is that any such process consists of four stages: the clarifying of the design task, the conceptual design, the embodiment design and the detailed design (cf. Pahl and Beitz (1996) ). In this paper I will not focus on such activities but rather on their direct outcomes which, in engineering design, are dubbed specifications.

There are a huge number of types of design specifications. There are cooking recipes, electrical schemes, elevation drawings, flow diagrams, musical scores, point paper designs, etc. Peter Simons and Charles Dement described the structure of the realm of designs by distinguishing between engineering, manufacturing and logistic bills for materials. When I speak in this paper about designs, I will be referring solely to those designs that correspond directly to engineering bills for material, or to those designs that specify the details of ready-to-use artefacts. Thus, the so-called manufacturing bills of materials and the logistic bills of materials are not designs in the sense used in this paper (cf. Simons and Dement (1996, pp. 267-268) ).

The variety of engineering bills of materials is, however, still enormous. What do all these designs have in common? What sort of mental structures, if any, are eligible for artefact design? What is the relation between the design of an artefact and the physical structure of the artefact?

Artefacts are products of human action. The human production of artefacts is something that is intimately linked to beliefs, desires and intentions. The representational content of these mental structures determines the relevant features of artefacts while co-determining certain phases of the production processes. In short, artefacts are design-based physical objects. In this paper I assume that this old Aristotelian view of artefacts is adequate also for contemporary kinds of artefacts, provided that we have a broad enough understand of the details of the picture.

Artefacts are designed and used by intentional agents. Every object capable of bringing about some state of affairs will be called an agent. If an agent has certain beliefs and wishes then it will be called an intentional agent. I assume that for every domain of artefacts, there is a community of intentional agents whose beliefs about the artefacts from that domain are reliable. I will assert that members of such a community are rational agents. In particular, if a rational agent believes that some artefact is designed to have certain features then her belief will be true.

What are designs? I will start with the classification problem: What is the ontological category of designs? As ordinary objects all artefacts have certain properties, take part in various processes, occur in events, etc. Thus, if we were asked to characterise them, we might come up with a set of sentences specifying the properties that the artefact has, the processes in which it takes part, etc. Every such sentence will refer to a state of affairs in which the artefact occurs. For example, specifying one feature of a resistor the sentence ‘This resistor has the resistance of 10 kΩ’ will refer to the state of affairs of the resistor having a resistance of 10 kΩ. The “fusion” of all states of affairs in which an object occurs may be called the ontic range of objects.

Artefacts are products of human activity that essentially involve mental components. Apparently, artefact designs somehow represent artefacts. If one says that x represents y, one might mean one of two things. First, one might wish to express the fact that x is an image of or mirrors y, in which case it is y that comes first and x that is presumed to imitate y. Here, it is x that is evaluated as a faithful or unfaithful representation of y. If x represents y in this sense, I will say that x represents ₁ y and call such a relation the relation of epistemic representation. Secondly, saying that x represents y one might wish to express the fact that y is an image of or mirrors x, in which case it is x that comes first and y that is supposed to imitate x. Here, it is y that is evaluated as a faithful or unfaithful representation of x. If x represents y in this sense, I will say that x represents ₂ y and call such a relation the relation of poietic representation. If x represents ₁ or ₂ y, I will still claim that x represents y. Admittedly, in claiming that artefact designs represent artefacts, I am asserting that the former represent ₂ the latter.

Usually there is nothing in the content of a representation of an entity that makes it a representation ₁ or a representation ₂ of that entity. It is a user of a given representation that determines that by using it either as an epistemic or poietic representation. Moreover, it is not so that any representation ₁ may be viewed as a representation ₂ ; however, any representation ₂ may become a representation ₁ . In our case it is a community of rational agents that determines precisely which representations are poietic representations of artefacts.

My claim that artefact designs represent ₂ artefacts is the weakest claim among all the claims based on the Aristotelian view of artefacts. In order to explain this metatheoretical remark, two additional qualifications of the relation of representation need to be given. I will say that x truthfully represents y iff every representational detail of x corresponds to some detail of y. I will also say that x adequately represents y iff every detail of y corresponds to some representational detail of x.

(1.1)

1. (At least) some designs truthfully represent ₂ some artefacts.
2. For any artefact x, no design adequately represents ₂ x.

Later on 1.1(i) will be strengthened to the “at most some” condition. 1.1(ii) accounts for the fact that human designs are products of finite minds that are unable to describe all the details of any their products. Consequently, a design of an artefact never specifies the whole ontic range of any one artefact.

Designs are like other representation of reality: they contain both objective and subjective components. An electrical plan is a depiction plotted in ink or chalk (the objective component of the design) by means of which a rational agent represents ₂ an electrical device (the subjective component of the design). Generally speaking, designs are physical objects of various kinds (inscriptions, drawings, pictures, sounds, etc.) that are considered by rational agents to be representations ₂ of other physical objects. With any artefact, the objective factor of its design may vary while the subjective factor remains constant. One might represent ₂ an electrical device by means of a drawing, a complex depiction or a series of sounds, but all these physical entities represent ₂ one and the same artefact. This means that the drawing, the depiction and the sounds may be perceived as representations ₂ of the artefact that represent ₂ it in the same way, in other words, as providing the same poietic information on that artefact. The following distinction would thus seem to be useful.

(1.2) An engineering specification of an artefact is a physical object considered by a rational agent to be a representation ₂ of the artefact.

(1.3) A design of an artefact is an entity by which means a rational agent considers different engineering specifications to be representations ₂ of the same artefact.

When a rational agent identifies by means of design y different engineering specifications z ₁ and z ₂ as representations ₂ of the same artefact, I will say that z ₁ and z ₂ (physically) support y.

The foregoing remarks should make it clear that every engineering specification supports at least one design. Assuming that designing is a rational activity, I stipulate that the relation of support is a function or that no engineering specification supports more than one design.

The design of an artefact partially determines what the artefact is like, that is to say, it partially determines the states of affairs in which the artefact is involved. ² This claim presupposes that artefact designs have a propositional structure, in other words, it presupposes that in principle any design might be supported by a complex inscription (i.e., a mereological sum of sentences ³ ). Obviously, designs are not sum totals of sentences because when a designer constructs a design she does not need to formulate a sentence and a great number of actual designs are not (and presumably will not be) supported in this way. However, since it is sufficient for a sentence to exist if the language of which this sentence is a part exists, I assume that for any engineering specification, there must be a mereological sum of sentences, the sum of which supports the same design as this specification. This assumption is not as solid as it looks if we concede that drawings or charts are (or consist of) sui generis sentences.

What, then, are designs? It is easy to observe that designs are neither engineering specifications nor mereological sums of engineering specifications. Regarding the former claim, many designs are supported by more than one engineering specification, none of which has the privileged position of being the engineering specification of a given artefact. Secondly, you can multiply engineering specifications, for instance, by photocopying them, without multiplying designs.

Thirdly, you can change or even destroy an engineering specification without changing the design that is supported by the engineering specification. As for the latter claim, it should be noticed that the mereological sum of a set of objects changes when one of the objects is changed. We cannot therefore change an engineering specification without changing the sum of the engineering specifications to which this engineering specification belongs. Consequently, if designs were mereological sums of engineering specifications, then any change in any engineering specification would precipitate a change in design. For similar reasons a design cannot be identified with a distributive set of engineering specifications.

In this paper I will explore the solution to the effect that artefact designs belong to the category of intentional states of affairs introduced by the Polish philosopher Roman Ingarden. Let me briefly sketch the main ideas of his conception (cf. Ingarden (1964; 1965) ).

2. States of affairs

If one slightly simplifies the issue, one might say that Ingarden distinguishes two different ontological categories of states of affairs: real and intentional. A real state of affairs may be defined as a part of the ontic range of an object. ⁴ For instance, if John is handsome, the fact that John is handsome is part of his ontic range since the property of being handsome inheres in John. Similarly, the fact that Paul hates Barbara and Mary runs are states of affairs. Knowing about such a state of affairs is part of the ontic range of an object, I know what the object is like, in what process it is involved or when it occurs. If a state of affairs x is a part of the ontic range of an object y, I will say that y occurs in x.

On the matter of intentional states of affairs, Ingarden says that due to its content every representational state of mind determines an intentional state of affairs. Derivatively, due to its meaning, every declarative sentence determines an intentional state of affairs. For example, if John believes that George W. Bush is a Catholic and if he discusses with a friend the impact of this fact on Bush’s international politics, then both refer to the same entity, but because John’s belief is false, no real state of affairs corresponds to it. Intentional entities, including intentional states of affairs, function as intermediate entities between mind (or language) and reality. Just as real objects occur in real states of affairs, so intentional objects occur in intentional states of affairs. ⁵ As a rough approximation, one might identify the category of intentional states of affairs with the category of propositions, provided that the latter are construed as mind-dependent entities. Therefore, the category of intentional states of affairs cannot be reduced to the category of sentences because two different sentences may determine the same intentional state of affairs, but one of the two may well cease to exist without the intentional state of affairs ceasing to exist. For a similar reason, the former category cannot be reduced to the category of representational states of mind.

What, then, is the relationship between the realm of real states of affairs and the realm of intentional states of affairs? Assume that an intentional agent formulates some kind of sentence. If the sentence is true, the intentional state of affairs determined by that sentence will mirror some actual state of affairs (i.e., a fact ) in the ontological universe. Ingarden adds that the intentional state of affairs and its actual counterpart share the same content, but differ in their mode of existence: actual states of affairs exist autonomously and intentional states of affairs exist heteronomously. He explains that existing autonomously involves having ontic foundations in itself while existing heteronomously means having ontic origins in something else. For example, the intentional state of affairs that George W. Bush is a Catholic has its ontic roots in John’s belief that George W. Bush is a Catholic.

If a representational state of mind or a declarative sentence determines an intentional state of affairs that corresponds to some real state of affairs, I will assert that the state of mind or the sentence represents (either epistemically or poietically) this real state of affairs. I will also claim that the respective intentional state of affairs represents the actual state of affairs. Similarly, I will say that intentional objects represent real objects.

I believe that given the foregoing explanation I am justified in identifying artefact designs with Ingarden’s intentional states of affairs. More precisely, artefact designs are construed here as structures composed of those intentional states of affairs that represent some real states of affairs. In order to erect my account of artefact designs on firm conceptual ground, I hereby propose the following formal theory on states of affairs which encompasses some ideas taken from the philosophical legacy of Roman Ingarden.

First Ingarden’s theory can be supplemented by the relation of parthood between states of affairs. As parts of ontic ranges of objects, states of affairs may be parts of one another. The state of affairs that John is handsome is also part of the state of affairs that he is handsome and shy. The state of affairs that John is an accountant is part of the state of affairs that he is an absent-minded accountant. To generalise, the state of affairs that p is part of the state of affairs that p and q. However, there are also non-conjunctive cases of parthood. For example, the state of affairs that John runs is part of the state of affairs that he is a fast runner.

The expression ‘ Occ (x, y)’ will mean that an object x occurs in a state of affairs y (or that y is a part of the ontic range of x ). The expression ‘ x≤y’ will mean that a state of affairs x is part of a state of affairs y.

The formal theory of states of affairs is defined in axioms 2.2-2.6, and 2.8 below. Since these axioms constitute fairly weak characteristics of the respective notions, the resulting theory might be called a minimal ontology of states of affairs. All axioms are to hold both for real and intentional entities.

Given the relation of occurrence we may define the notions of object (2.1(i)) and of states of affairs (2.1(ii)):

(2.1)

1. Obj (x) = ∃ y Occ (x, y).
2. Soa (x) = ∃y Occ (y, x). ⁶

The following two axioms express the categorical constraints on objects and states of affairs.

(2.2) Obj (x) = ¬ Soa (x).

(2.3) x≤y → Soa (x) ∧ Soa (y).

Like Roberto Casati and Achille Varzi, I endorse the lexical principle to the effect that any relation of parthood is a partial order ( Casati and Varzi 1999, p. 33). I express this principle in terms of axioms 2.4-2.6:

(2.4) Soa (x) → x≤x.

(2.5) x≤y ∧ y≤x ®→ x=y.

(2.6) x≤ y ∧ y≤z → x≤z.

The expression ‘x<y’ will mean that a state of affairs x is a proper part of a state of affairs y.

(2.7) x<y = x≤y ∧ x≠y.

It follows from the axioms and 2.7 that the proper parthood is irreflexive, asymmetric and transitive.

Given the metaphor of ontic range it would seem obvious that if a state of affairs x is part of a state of affairs y and an object z occurs in x (i.e., if x is part of the ontic range of z ), then z will also occur in y:

(2.8) If x≤y, then ∀z [ Occ (z, x) → Occ (z, y) ]. ⁷

3. Designs as states of affairs

I claim that designs are intentional states of affairs. What does this claim mean? Assume that a rational agent has designed an artefact. I argued above that:

1. the resulting design represents ₂ part of the ontic range of the designed artefact,
2. there is a mereological sum of sentences supporting that design.

Every sentence from the sum referred to in (ii) creates an intentional state of affairs. Every such state represents a part of the ontic range referred to in (i). Let X be the set of these intentional states of affairs. Because of the unity of the designer’s intentions, the design is also a unified entity. Given the above theory of states of affairs, the simplest way to represent the latter unity is to require that the design be the state of affairs that is the least upper bound of X with respect to ≤.

‘A’ will denote a set of artefacts. Let L _A be a language whose conceptual resources are adequate for speaking about the artefacts from A. Then S _A becomes the set of all intentional states of affairs created by the sentences from L _A .

(3.1) For every artefact x, there is a non-empty set X⊆S _A so that the least upper bound of X is a design of x.

How many designs does an artefact have? At first sight, the answer seems straightforward: every artefact has exactly one design. Observe, however, that an artefact may be part of another artefact and that a design of the latter may not specify all details of the former. A diode is a part of a power supply. The design of the diode that is part of the broader power supply design specifies only two parts of the diode: the anode and the cathode. Still, a more detailed design of the diode, for instance the design found in a handbook on general electronics, also mentions a semi-conductor junction between the anode and cathode. Consequently, the diode has at least two designs. Consider also another case. Assume, for argument’s sake, that political organisations are artefacts. The European Union ‘design’ lists the Republic of Poland as one of its parts and the provinces of Poland as parts of Poland. Nevertheless, the design does not mention that the many localities of Poland are parts of the EU. Still, the design of the Republic of Poland, as established in administrative Polish law, does mention these localities as parts of Poland. It therefore appears that ordinary parlance does allow us to admit that the same artefact has different designs. ⁸ Strictly speaking, in their professional activities artefact designers seem to represent ₂ one artefact by means of different representations ₂ . One, usually the most specific, representation ₂ is created before the artefact is produced. Other, less specific, representations ₂ are created when the artefact is used as a component of or as a tool for other artefacts. The fact that the most specific design is not deployed in the latter case is not a contingent matter but rather a consequence of the bounded rationality involved in engineering practice. In most cases, if a design x of a technical artefact were to contain the most specific designs of the components of the artefact, the design x would be an extremely complex representation. If, but only if, engineers were intentional agents with unlimited cognitive capacities, they might be able to ignore the complexity of their products. Later on I will also illustrate that the fact that one artefact has more than one design makes it possible to draw a useful distinction between artefact tokens and artefact types.

Given my identification of designs with intentional states of affairs, the most adequate approximation of the relation of specificity between designs is the relation of proper parthood between states of affairs.

(3.2) A design y ₁ is less specific than a design y ₂ iff y ₁ <y ₂ .

I claim that the set of all designs associated with a given artefact has its greatest and least elements with respect to <. The existence of the former guarantees that every artefact is uniquely determined by its design, as far as its physical structure is concerned. The existence of the latter guarantees that there is an objective rationale for artefact token identification. Let me explain the latter claim in more detail.

Your new laptop has some dead pixels on the display. You want to reclaim it, so you visit the technical support unit. When you hear there that the laptop on the shelf is the same as your laptop, you do not start complaining that the technical support unit breaches the law of indiscernibility of identical artefacts. You presume that they mean that your laptop is a copy of the laptop on the shelf. We know that artefacts exist, so to speak, in copies. What we do not know is the conditions under which one artefact may be a copy of another.

It turns out that your second laptop is even worse than the first one. You are forced to replace one part after another. When does the original laptop on your desk cease to exist because of this replacement process? We know that in the course of time artefacts undergo various changes. What we do not know is the conditions under which artefacts preserve their identity over the course of time or cease to exist.

These and similar problems are more tractable if we reformulate them in terms of artefact tokens and artefact types. Then, instead of pursuing the question of when one artefact becomes a copy of another artefact, we pursue the question of when one artefact token is a token of the same artefact type as another artefact token. For example, the question ‘Is my laptop a copy of that laptop on the shelf?’ is superseded by the question ‘Is this token of a laptop a token of the same artefact type as that token of a laptop?’ Instead of pursuing the question of under what conditions artefacts endure in terms of time, we pursue the question of when an object is a token of a given artefact type. For example, the question ‘Does my laptop survive (i.e., preserve its identity despite) the replacement of its battery B with a new battery B ?’ is substituted by the question ‘Will the replacement of B with B in this artefact token lead to a change in its artefact type?’ or by the question ‘Is this artefact token with the old battery B of the same artefact type as the artefact token with the new battery B’ ?’

An artefact token is an artefact in the ordinary sense of the word. Artefact tokens are physical entities located in time and space. It is artefact tokens that we use as writing tools. They have certain properties, participate in processes and initiate events. One artefact token may be a copy of another. Within the ontological framework sketched above, one may say that artefact tokens are real objects and that their ontic ranges consist of real states of affairs. On the other hand, an artefact type is an abstract object like a universal. An artefact type gathers the features common to a group of artefact tokens. Artefact types are not located in time or space, therefore you cannot write philosophical papers with them; nonetheless, artefact types are useful conceptual devices. When I learn about the significant features of some artefact token, I may limit my investigation to the features specified by the artefact type of this token. When I notice that two artefact tokens x and y are similar in relevant respects, I may express my observation by stating that x is of the same artefact type as y. When I wish to make a general observation about a group of artefact tokens, I may express that by saying that the respective artefact type exhibits the observed regularity. In short, artefact types represent artefact tokens. Given the ontological framework sketched above, one may conclude that artefact types are intentional objects.

By now it should be obvious that the most specific design exhaustively determines the ontic range of the respective artefact type but the artefact tokens of such a type are not thereby completely determined. However, we must not compare the ontic ranges of artefacts types with the most specific designs. Since we have to allow for the fact that some artefact tokens malfunction, we must not say that two artefact tokens are tokens of the same artefact type if their ontic ranges are only represented by the same most specific design. Even if an artefact token loses some of the features specified in its most specific design, the token may still “retain its artefact type”. We ought to base the identity of artefact tokens on less specific designs and the most natural candidates are the respective least specific designs. If every artefact has its least specific design, then we may say that two artefact tokens are tokens of the same artefact type if their ontic ranges are represented by the same least specific design. I will thus compare the ontic ranges of artefact types with the least specific designs of artefacts.

This then places us in a position to solve our initial puzzles, the puzzle of copies and the puzzle of replacement. A physical object x is an artefact token of an artefact type y iff the least specific design of y represents ₂ a part of the actual ontic range of x. When x is an artefact token of y, y will be termed an artefact type of x. A physical object x ₁ is a token of the same artefact type as a physical object x ₂ iff there is an artefact type in which both x ₁ and x ₂ are tokens. Observe that if the relation of being an artefact token is a function (i.e., if x is an artefact token both of artefact type y ₁ and of artefact type y ₂ , then y ₁ =y ₂ ), then the relation denoted by the expression ‘... is of the of the same artefact type as ...’ is transitive. ⁹

Consequently, my laptop is a copy of that laptop on the shelf iff there is evidence of the least specific artefact design representing parts of the ontic ranges of both laptops. My laptop preserves its identity as long as its least specific design represents some part of its ontic range or if the least specific design represents some part of the ontic range of the laptop with B' rather than B, in which case the laptop survives the replacement of B with B'. On the other hand, if the design at stake represents no part of the ontic range of the laptop with B', then the replacement process puts an end to the laptop.

Let the expression ‘ design (y, x)’ abbreviate the expression ‘a state of affairs y is a design of an object x’. Since designs are intentional states of affairs, the intended domain of the following formal theory of designs contains only intentional entities: intentional objects and intentional states of affairs.

I define artefacts types as “design-based intentional objects”:

(3.3) Art (x) = ∃ y design (y, x).

I claim in this paper that designs are (intentional) states of affairs representing objects.

(3.4) design (y, x) → Soa (y) ∧ Obj (x).

The most specific design of an artefact x will be called the full design of x and the least specific design will be called the minimal design of x. The fact that y is the full design of x will be denoted as Design (y, x). The fact that y is the minimal design of x will be denoted as design ₀ (y, x).

(3.5)

1. Design (y, x) = design (y, x) ∧ ∀z [ design (z, x) → z≤y ],
2. design ₀ (y, x) = design (y, x) ∧ ∀z [ design (z, x) → y≤z ].

3.6 is the conclusion of the foregoing argument.

(3.6) Art (x) → ∃ y ₁ , y ₂ [ Design (y ₁ , x) ∧ design ₀ (y ₂ , x) ].

It follows from 3.5 and 3.6 that the full design of x and the minimal design of x are unique, therefore I will denote them as Design (x) and design ₀ (x).

The notion of minimal design is the objective basis for artefact identification. This means that if x is an artefact type then the minimal design of x will be part of every state of affairs in which x occurs:

(3.7) Occ (x, y) ∧ design ₀ (z, x) → z≤y.

The above axioms do not guarantee that artefact designs are not circular. There are at least two kinds of circularity at stake. The first type is more straightforward. Both artefacts and non-artefacts may occur in artefact designs but on pain of infinite regress I assume that no artefact occurs in its own design.

(3.8) Design (y, x) → ¬ Occ (x, y).

3.8 does not proscribe the design supported by the sentence ‘the hammer x consists of the haft y and …’, but it does proscribe the design supported by the sentence ‘the hammer x consists of the hammer x and …’. Incidentally, 3.8 is related to the special sense of the expression ‘A design of an artefact determines the ontic range of the artefact’. Ultimately if y is a design of x , then y does not specify in what states of affairs x occurs but rather the states of affairs in which the objects which compose x occur.

The second type of circularity is more complex. Assume that a design of an artefact x ₁ is less specific than a design of an artefact x ₂ and that a design of x ₂ is less specific than a design of x ₁ . If we conceded that all artefacts have just one design, such a case would be excluded by the asymmetry of the relation of being less specific than (i.e., relation <). However, since we allow some artefacts to have more than one design, the situation depicted in Fig. 1 is possible.

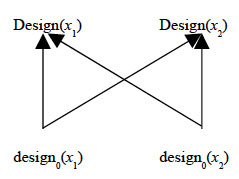
Figure 1

In this case it seems that x ₁ is a proper part of x ₂ (because a design of x ₁ is a proper part of a design of x ₂ ) and x ₂ is a proper part of x ₁ (because a design of x ₂ is a proper part of a design of x ₁ ), which is an absurd conclusion. In order to exclude such cases, I introduce axiom 3.9:

(3.9) design ₀ (x ₁ ) < Design (x ₂ ) ∧ design ₀ (x ₂ ) < Design (x ₁ ) → x ₁ =x ₂ .

Of course 3.9 does not eliminate all cases of design circularity. For instance, 3.9 allows the following situation to occur:

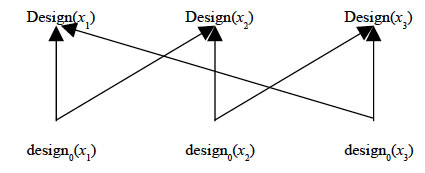
Figure 2

However, in order to exclude all such cases we either need to denumerably introduce many first-order axioms or to resort to a second-order theory.

The identifying of minimal designs with ontic ranges of artefact types means that artefacts types with the same minimal designs become identical. Needless to say, if two artefact types have the same non-minimal design, then they are identical as well. So if x ₁ and x ₂ are artefact types, then the following claim is valid:

(3.10) design (y, x ₁ ) ∧ design (y, x ₂ ) → x ₁ =x ₂ .

Axioms 2.2-2.6, 2.8, 3.4, 3.6-3.10 and definitions 2.1, 2.7, 3.3, and 3.5 constitute my formal theory of artefact designs.

5. Conclusions

In this paper I have provided an ontological framework for the notion of artefact design, which is understood to be an outcome of designing activity. The minimal ontological commitments of the design discourse were revealed and formalised. I argued that given the above construal of this notion, we should account for the fact that some artefacts have more than one design. Finally, I introduced a series of constraints in order to exclude any “irrational” cases of design.

References

Endnotes

¹ One of the numerous examples of the theory of axiomatic design, developed by N. P. Suh, which provides axioms that govern the design process, e.g., “Minimize the information content of the design”. See Suh (2001).

² The precise significance of this claim is expressed by the following implication: if a design of an artefact determines that the artefact is such and such, then it is the case that the artefact is such and such. The claim does not entail the converse of that implication. At present I ignore the distinction between ‘is such and such’ and ‘is designed to be such and such’.

³ In what follows, the term ‘sentence’ will refer to sentence-tokens, i.e., inscriptions or series of phones.

⁴ Actually we should distinguish the inner ontic range of an object, i.e., its ontic structure, and the outer range of the object. The former corresponds to the notion of essence or nature; the latter to the notion of accident.

⁵ Intentional objects are in this sense different from intentional objects in the sense defined in Baker’s paper included in this issue.

⁶ Although the minimal ontology of states of affairs refers to two kinds of entities: objects and states of affairs, the ontology is not a many-sorted theory. Subsequently, all variables range between the same set of entities.

⁷ Since in this paper I only use my theory of states of affairs as a tool to grasp the ontological properties of artefact designs, I do not compare it with other formal accounts of states of affairs. I decided not to employ these accounts either because the complexity of their formalism is too great for the present purposes (e.g., Barwise and Perry (1983)) or because they involve more philosophical commitments than my minimal proposal permits (e.g., Armstrong (1997)).

⁸ In Hubka and Eder (1988) it is claimed that technical artefacts are represented by different engineering specifications.

⁹ Needless to say, the notion of least specific design does not solve all identity puzzles because we cannot distinguish different tokens of the same type on the basis of this notion.